User:Tomruen/Uniform 5-polytope

Graphs of regular and uniform polytopes.

5-simplex	Rectified 5-simplex		Truncated 5-simplex
Cantellated 5-simplex	Runcinated 5-simplex		Stericated 5-simplex
5-orthoplex	Truncated 5-orthoplex		Rectified 5-orthoplex
Cantellated 5-orthoplex		Runcinated 5-orthoplex
Cantellated 5-cube	Runcinated 5-cube		Stericated 5-cube
5-cube	Truncated 5-cube		Rectified 5-cube
5-demicube		Truncated 5-demicube
Cantellated 5-demicube		Runcinated 5-demicube

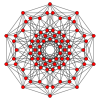
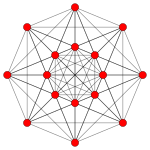
In geometry, a uniform 5-polytope is a five-dimensional uniform polytope. By definition, a uniform 5-polytope is vertex-transitive and constructed from uniform 4-polytope facets.

The complete set of convex uniform 5-polytopes has not been determined, but most can be made as Wythoff constructions from a small set of symmetry groups. These construction operations are represented by the permutations of rings of the Coxeter diagrams.

History of discovery

Regular polytopes: (convex faces)
- 1852: Ludwig Schläfli proved in his manuscript Theorie der vielfachen Kontinuität that there are exactly 3 regular polytopes in 5 or more dimensions.
Convex semiregular polytopes: (Various definitions before Coxeter's uniform category)
- 1900: Thorold Gosset enumerated the list of nonprismatic semiregular convex polytopes with regular facets (convex regular polychora) in his publication On the Regular and Semi-Regular Figures in Space of n Dimensions.^[1]
Convex uniform polytopes:
- 1940: The search was expanded systematically by H.S.M. Coxeter in his publication Regular and Semi-Regular Polytopes.
Nonregular uniform star polytopes: (similar to the nonconvex uniform polyhedra)

Regular 5-polytopes

Regular 5-polytopes can be represented by the Schläfli symbol {p,q,r,s}, with s {p,q,r} polychoral facets around each face. There are exactly three such regular polytopes, all convex:

{3,3,3,3} - 5-simplex
{4,3,3,3} - 5-cube
{3,3,3,4} - 5-orthoplex

There are no nonconvex regular polytopes in 5 or more dimensions.

Convex uniform 5-polytopes

There are 104 known convex uniform 5-polytopes, plus a number of infinite families of duoprism prisms, and polygon-polyhedron duoprisms. All except the grand antiprism prism are based on Wythoff constructions, reflection symmetry generated with Coxeter groups.

Reflection families

Coxeter diagram correspondences between families and higher symmetry within diagrams. Nodes of the same color in each row represent identical mirrors. Black nodes are not active in the correspondence.

The 5-simplex is the regular form in the A₅ family. The 5-cube and 5-orthoplex are the regular forms in the B₅ family. The bifurcating graph of the D₆ family contains the pentacross, as well as a 5-demicube which is an alternated 5-cube.

Fundamental families

#	Coxeter group
1	A₅	[3⁴]
2	B₅	[4,3³]
3	D₅	[3^2,1,1]

Uniform prismsThere are 5 finite categorical uniform prismatic families of polytopes based on the nonprismatic uniform 4-polytopes:

#	Coxeter groups
1	A₄ × A₁	[3,3,3,2]
2	B₄ × A₁	[4,3,3,2]
3	F₄ × A₁	[3,4,3,2]
4	H₄ × A₁	[5,3,3,2]
5	D₄ × A₁	[3^1,1,1,2]

There is one infinite family of 5-polytopes based on prisms of the uniform duoprisms {p}×{q}×{ }:

Coxeter groups		Coxeter diagram
I₂(p) × I₂(q) × A₁	[p,2,q,2]

Uniform duoprisms

There are 3 categorical uniform duoprismatic families of polytopes based on Cartesian products of the uniform polyhedra and regular polygons: {q,r}×{p}:

#	Coxeter groups
1	A₃ × I₂(p)	[3,3,2,p]
2	B₃ × I₂(p)	[4,3,2,p]
3.	H₃ × I₂(p)	[5,3,2,p]

Enumerating the convex uniform 5-polytopes

Simplex family: A₅ [3⁴]
- 19 uniform 5-polytopes
Hypercube/Orthoplex family: BC₅ [4,3³]
- 31 uniform 5-polytopes
Demihypercube D₅/E₅ family: [3^2,1,1]
- 23 uniform 5-polytopes (8 unique)
Prisms and duoprisms:
- 56 uniform 5-polytope (46 unique) constructions based on prismatic families: [3,3,3]×[ ], [4,3,3]×[ ], [5,3,3]×[ ], [3^1,1,1]×[ ].
- One non-Wythoffian - The grand antiprism prism is the only known non-Wythoffian convex uniform 5-polytope, constructed from two grand antiprisms connected by polyhedral prisms.

That brings the tally to: 19+31+8+46+1=105

In addition there are:

Infinitely many uniform 5-polytope constructions based on duoprism prismatic families: [p]×[q]×[ ].
Infinitely many uniform 5-polytope constructions based on duoprismatic families: [3,3]×[p], [4,3]×[p], [5,3]×[p].

The A₅ family

There are 19 forms based on all permutations of the Coxeter diagrams with one or more rings. (16+4-1 cases)

They are named by Norman Johnson from the Wythoff construction operations upon regular 5-simplex (hexateron).

The A₅ family has symmetry of order 720 (6 factorial). 7 of the 19 figures, with symmetrically ringed Coxeter diagrams have doubled symmetry, order 1440.

The coordinates of uniform 5-polytopes with 5-simplex symmetry can be generated as permutations of simple integers in 6-space, all in hyperplanes with normal vector (1,1,1,1,1,1).

See symmetry graphs: List of A5 polytopes

#	Base point	Johnson naming system Bowers name and (acronym) Coxeter diagram	k-face element counts					Vertex figure	Facet counts by location: [3,3,3,3]
#	Base point		4	3	2	1	0	Vertex figure	[3,3,3] (6)	[3,3,2] (15)	[3,2,3] (20)	[2,3,3] (15)	[3,3,3] (6)
1	(0,0,0,0,0,1) or (0,1,1,1,1,1)	5-simplex hexateron (hix)	6	15	20	15	6	{3,3,3}	(5) {3,3,3}	-	-	-	-
2	(0,0,0,0,1,1) or (0,0,1,1,1,1)	Rectified 5-simplex rectified hexateron (rix)	12	45	80	60	15	t{3,3}×{ }	(4) r{3,3,3}	-	-	-	(2) {3,3,3}
3	(0,0,0,0,1,2) or (0,1,2,2,2,2)	Truncated 5-simplex truncated hexateron (tix)	12	45	80	75	30	Tetrah.pyr	(4) t{3,3,3}	-	-	-	(1) {3,3,3}
4	(0,0,0,1,1,2) or (0,1,1,2,2,2)	Cantellated 5-simplex small rhombated hexateron (sarx)	27	135	290	240	60	prism-wedge	(3) rr{3,3,3}	-	-	(1) × { }×{3,3}	(1) r{3,3,3}
5	(0,0,0,1,2,2) or (0,0,1,2,2,2)	Bitruncated 5-simplex bitruncated hexateron (bittix)	12	60	140	150	60		(3) 2t{3,3,3}	-	-	-	(2) t{3,3,3}
6	(0,0,0,1,2,3) or (0,1,2,3,3,3)	Cantitruncated 5-simplex great rhombated hexateron (garx)	27	135	290	300	120		tr{3,3,3}	-	-	× { }×{3,3}	t{3,3,3}
7	(0,0,1,1,1,2) or (0,1,1,1,2,2)	Runcinated 5-simplex small prismated hexateron (spix)	47	255	420	270	60		(2) t_0,3{3,3,3}	-	(3) {3}×{3}	(3) × { }×r{3,3}	(1) r{3,3,3}
8	(0,0,1,1,2,3) or (0,1,2,2,3,3)	Runcitruncated 5-simplex prismatotruncated hexateron (pattix)	47	315	720	630	180		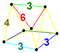t_0,1,3{3,3,3}	-	× {6}×{3}	× { }×r{3,3}	rr{3,3,3}
9	(0,0,1,2,2,3) or (0,1,1,2,3,3)	Runcicantellated 5-simplex prismatorhombated hexateron (pirx)	47	255	570	540	180		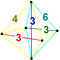t_0,1,3{3,3,3}	-	{3}×{3}	× { }×t{3,3}	2t{3,3,3}
10	(0,0,1,2,3,4) or (0,1,2,3,4,4)	Runcicantitruncated 5-simplex great prismated hexateron (gippix)	47	315	810	900	360	Irr.5-cell	t_0,1,2,3{3,3,3}	-	× {3}×{6}	× { }×t{3,3}	rr{3,3,3}
11	(0,1,1,1,2,3) or (0,1,2,2,2,3)	Steritruncated 5-simplex celliprismated hexateron (cappix)	62	330	570	420	120		t{3,3,3}	× { }×t{3,3}	× {3}×{6}	× { }×{3,3}	t_0,3{3,3,3}
12	(0,1,1,2,3,4) or (0,1,2,3,3,4)	Stericantitruncated 5-simplex celligreatorhombated hexateron (cograx)	62	480	1140	1080	360		tr{3,3,3}	× { }×tr{3,3}	× {3}×{6}	× { }×rr{3,3}	t_0,1,3{3,3,3}

#	Base point	Johnson naming system Bowers name and (acronym) Coxeter diagram	k-face element counts					Vertex figure	Facet counts by location: [3,3,3,3]
#	Base point		4	3	2	1	0	Vertex figure	[3,3,3] (6)	[3,3,2] (15)	[3,2,3] (20)	[2,3,3] (15)	[3,3,3] (6)
13	(0,0,0,1,1,1)	Birectified 5-simplex dodecateron (dot)	12	60	120	90	20	{3}×{3}	(3) r{3,3,3}	-	-	-	(3) r{3,3,3}
14	(0,0,1,1,2,2)	Bicantellated 5-simplex small birhombated dodecateron (sibrid)	32	180	420	360	90		(2) rr{3,3,3}	-	(8) {3}×{3}	-	(2) rr{3,3,3}
15	(0,0,1,2,3,3)	Bicantitruncated 5-simplex great birhombated dodecateron (gibrid)	32	180	420	450	180		tr{3,3,3}	-	{3}×{3}	-	tr{3,3,3}
16	(0,1,1,1,1,2)	Stericated 5-simplex small cellated dodecateron (scad)	62	180	210	120	30	Irr.16-cell	(1) {3,3,3}	(4) × { }×{3,3}	(6) {3}×{3}	(4) × { }×{3,3}	(1) {3,3,3}
17	(0,1,1,2,2,3)	Stericantellated 5-simplex small cellirhombated dodecateron (card)	62	420	900	720	180		rr{3,3,3}	× { }×rr{3,3}	{3}×{3}	× { }×rr{3,3}	rr{3,3,3}
18	(0,1,2,2,3,4)	Steriruncitruncated 5-simplex celliprismatotruncated dodecateron (captid)	62	450	1110	1080	360		t_0,1,3{3,3,3}	× { }×t{3,3}	{6}×{6}	× { }×t{3,3}	t_0,1,3{3,3,3}
19	(0,1,2,3,4,5)	Omnitruncated 5-simplex great cellated dodecateron (gocad)	62	540	1560	1800	720	Irr. {3,3,3}	(1) t_0,1,2,3{3,3,3}	(1) × { }×tr{3,3}	(1) {6}×{6}	(1) × { }×tr{3,3}	(1) t_0,1,2,3{3,3,3}

The B₅ family

The B₅ family has symmetry of order 3840 (5!×2⁵).

This family has 2⁵−1=31 Wythoffian uniform polytopes generated by marking one or more nodes of the Coxeter diagram.

For simplicity it is divided into two subgroups, each with 12 forms, and 7 "middle" forms which equally belong in both.

The 5-cube family of 5-polytopes are given by the convex hulls of the base points listed in the following table, with all permutations of coordinates and sign taken. Each base point generates a distinct uniform 5-polytope. All coordinates correspond with uniform 5-polytopes of edge length 2.

See symmetry graph: List of B5 polytopes

#	Base point	Name Coxeter diagram	Element counts					Vertex figure	Facet counts by location: [4,3,3,3]
#	Base point	Name Coxeter diagram	4	3	2	1	0	Vertex figure	[4,3,3] (10)	[4,3,2] (40)	[4,2,3] (80)	[2,3,3] (80)	[3,3,3] (32)
1	(0,0,0,0,1)√2	5-orthoplex (tac) (Quadrirectified 5-cube)	32	80	80	40	10	{3,3,4}	{3,3,3}	-	-	-	-
2	(0,0,0,1,1)√2	Rectified 5-orthoplex (rat) (Trirectified 5-cube)	42	240	400	240	40	{ }×{3,4}	{3,3,4}	-	-	-	r{3,3,3}
3	(0,0,0,1,2)√2	Truncated 5-orthoplex (tot) (Quadritruncated 5-cube)	42	240	400	280	80	(Octah.pyr)	t{3,3,3}	{3,3,3}	-	-	-
4	(0,0,1,1,1)√2	Birectified 5-cube (nit) (Birectified 5-orthoplex)	42	280	640	480	80	{4}×{3}	r{3,3,4}	-	-	-	r{3,3,3}
5	(0,0,1,1,2)√2	Cantellated 5-orthoplex (sart) (Tricantellated 5-cube)	82	640	1520	1200	240	Prism-wedge	r{3,3,4}	{ }×{3,4}	-	-	rr{3,3,3}
6	(0,0,1,2,2)√2	Bitruncated 5-orthoplex (bittit) (tritruncated 5-cube)	42	280	720	720	240		t{3,3,4}	-	-	-	2t{3,3,3}
7	(0,0,1,2,3)√2	Cantitruncated 5-orthoplex (gart) (tricantitruncated 5-orthoplex)	82	640	1520	1440	480		rr{3,3,4}	{ }×r{3,4}	{6}×{4}	-	t_0,1,3{3,3,3}
8	(0,1,1,1,1)√2	Rectified 5-cube (rit)	42	200	400	320	80	{3,3}×{ }	r{4,3,3}	-	-	-	{3,3,3}
9	(0,1,1,1,2)√2	Runcinated 5-orthoplex (spat)	162	1200	2160	1440	320		r{4,3,3}	-	{3}×{4}		t_0,3{3,3,3}
10	(0,1,1,2,2)√2	Bicantellated 5-cube (sibrant) (Bicantellated 5-orthoplex)	122	840	2160	1920	480		rr{4,3,3}	-	{4}×{3}	-	rr{3,3,3}
11	(0,1,1,2,3)√2	Runcitruncated 5-orthoplex (pattit)	162	1440	3680	3360	960		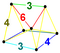rr{3,3,4}	{ }×r{3,4}	{6}×{4}	-	t_0,1,3{3,3,3}
12	(0,1,2,2,2)√2	Bitruncated 5-cube (pattin)	42	280	720	800	320		2t{4,3,3}	-	-	-	t{3,3,3}
13	(0,1,2,2,3)√2	Runcicantellated 5-orthoplex (pirt)	162	1200	2960	2880	960		{ }×t{3,4}	2t{3,3,4}	{3}×{4}	-	t_0,1,3{3,3,3}
14	(0,1,2,3,3)√2	Bicantitruncated 5-cube (gibrant) (Bicantitruncated 5-orthoplex)	122	840	2160	2400	960		rr{4,3,3}	-	{4}×{3}	-	rr{3,3,3}
15	(0,1,2,3,4)√2	Runcicantitruncated 5-orthoplex (gippit)	162	1440	4160	4800	1920		tr{3,3,4}	{ }×t{3,4}	{6}×{4}	-	t_0,1,2,3{3,3,3}
16	(1,1,1,1,1)	5-cube (pent)	10	40	80	80	32	{3,3,3}	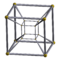{4,3,3}	-	-	-	-
17	(1,1,1,1,1) + (0,0,0,0,1)√2	Stericated 5-cube (scant) (Stericated 5-orthoplex)	242	800	1040	640	160	Tetr.antiprm	{4,3,3}	{4,3}×{ }	{4}×{3}	{ }×{3,3}	{3,3,3}
18	(1,1,1,1,1) + (0,0,0,1,1)√2	Runcinated 5-cube (span)	202	1240	2160	1440	320		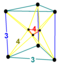t_0,3{4,3,3}	-	{4}×{3}	{ }×r{3,3}	{3,3,3}
19	(1,1,1,1,1) + (0,0,0,1,2)√2	Steritruncated 5-orthoplex (cappin)	242	1520	2880	2240	640		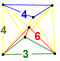t_0,3{3,3,4}	{ }×{4,3}	-	-	t{3,3,3}
20	(1,1,1,1,1) + (0,0,1,1,1)√2	Cantellated 5-cube (sirn)	122	680	1520	1280	320	Prism-wedge	rr{4,3,3}	-	-	{ }×{3,3}	r{3,3,3}
21	(1,1,1,1,1) + (0,0,1,1,2)√2	Stericantellated 5-cube (carnit) (Stericantellated 5-orthoplex)	242	2080	4720	3840	960		rr{4,3,3}	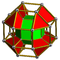rr{4,3}×{ }	{4}×{3}	{ }×rr{3,3}	rr{3,3,3}
22	(1,1,1,1,1) + (0,0,1,2,2)√2	Runcicantellated 5-cube (prin)	202	1240	2960	2880	960		t_0,1,3{4,3,3}	-	{4}×{3}	{ }×t{3,3}	2t{3,3,3}
23	(1,1,1,1,1) + (0,0,1,2,3)√2	Stericantitruncated 5-orthoplex (cogart)	242	2320	5920	5760	1920		{ }×rr{3,4}	t_0,1,3{3,3,4}	{6}×{4}	{ }×t{3,3}	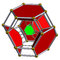tr{3,3,3}
24	(1,1,1,1,1) + (0,1,1,1,1)√2	Truncated 5-cube (tan)	42	200	400	400	160	Tetrah.pyr	t{4,3,3}	-	-	-	{3,3,3}
25	(1,1,1,1,1) + (0,1,1,1,2)√2	Steritruncated 5-cube (cappin)	242	1600	2960	2240	640		t{4,3,3}	t{4,3}×{ }	{8}×{3}	{ }×{3,3}	t_0,3{3,3,3}
26	(1,1,1,1,1) + (0,1,1,2,2)√2	Runcitruncated 5-cube (pattin)	202	1560	3760	3360	960		t_0,1,3{4,3,3}	{ }×t{4,3}	{6}×{8}	{ }×t{3,3}	t_0,1,3{3,3,3}]]
27	(1,1,1,1,1) + (0,1,1,2,3)√2	Steriruncitruncated 5-cube (captint) (Steriruncitruncated 5-orthoplex)	242	2160	5760	5760	1920		t_0,1,3{4,3,3}	t{4,3}×{ }	{8}×{6}	{ }×t{3,3}	t_0,1,3{3,3,3}
28	(1,1,1,1,1) + (0,1,2,2,2)√2	Cantitruncated 5-cube (girn)	122	680	1520	1600	640		tr{4,3,3}	-	-	{ }×{3,3}	t{3,3,3}
29	(1,1,1,1,1) + (0,1,2,2,3)√2	Stericantitruncated 5-cube (cogrin)	242	2400	6000	5760	1920		tr{4,3,3}	tr{4,3}×{ }	{8}×{3}	{ }×t_0,2{3,3}	t_0,1,3{3,3,3}
30	(1,1,1,1,1) + (0,1,2,3,3)√2	Runcicantitruncated 5-cube (gippin)	202	1560	4240	4800	1920		t_0,1,2,3{4,3,3}	-	{8}×{3}	{ }×t{3,3}	tr{3,3,3}
31	(1,1,1,1,1) + (0,1,2,3,4)√2	Omnitruncated 5-cube (gacnet) (omnitruncated 5-orthoplex)	242	2640	8160	9600	3840	Irr. {3,3,3}	tr{4,3}×{ }	tr{4,3}×{ }	{8}×{6}	{ }×tr{3,3}	t_0,1,2,3{3,3,3}

The D₅ family

The D₅ family has symmetry of order 1920 (5! x 2⁴).

This family has 23 Wythoffian uniform polyhedra, from 3x8-1 permutations of the D₅ Coxeter diagram with one or more rings. 15 (2x8-1) are repeated from the B₅ family and 8 are unique to this family.

See symmetry graphs: List of D5 polytopes

#	Coxeter diagram Schläfli symbol symbols Johnson and Bowers names	Element counts					Vertex figure	Facets by location: [3^1,2,1]
#		4	3	2	1	0	Vertex figure	[3,3,3] (16)	[3^1,1,1] (10)	[3,3]×[ ] (40)	[ ]×[3]×[ ] (80)	[3,3,3] (16)
51	= h{4,3,3,3}, 5-demicube Hemipenteract (hin)	26	120	160	80	16	t₁{3,3,3}	{3,3,3}	t₀(1₁₁)	-	-	-
52	= h₂{4,3,3,3}, cantic 5-cube Truncated hemipenteract (thin)	42	280	640	560	160
53	= h₃{4,3,3,3}, runcic 5-cube Small rhombated hemipenteract (sirhin)	42	360	880	720	160
54	= h₄{4,3,3,3}, steric 5-cube Small prismated hemipenteract (siphin)	82	480	720	400	80
55	= h_2,3{4,3,3,3}, runcicantic 5-cube Great rhombated hemipenteract (girhin)	42	360	1040	1200	480
56	= h_2,4{4,3,3,3}, stericantic 5-cube Prismatotruncated hemipenteract (pithin)	82	720	1840	1680	480
57	= h_3,4{4,3,3,3}, steriruncic 5-cube Prismatorhombated hemipenteract (pirhin)	82	560	1280	1120	320
58	= h_2,3,4{4,3,3,3}, steriruncicantic 5-cube Great prismated hemipenteract (giphin)	82	720	2080	2400	960

Uniform prismatic forms

There are 5 finite categorical uniform prismatic families of polytopes based on the nonprismatic uniform 4-polytopes:

A₄ × A₁

This prismatic family has 9 forms:

The A₁ x A₄ family has symmetry of order 240 (2*5!).

#	Coxeter diagram and Schläfli symbols Name	Element counts
#	Coxeter diagram and Schläfli symbols Name	Facets	Cells	Faces	Edges	Vertices
59	= {3,3,3}×{ } 5-cell prism	7	20	30	25	10
60	= r{3,3,3}×{ } Rectified 5-cell prism	12	50	90	70	20
61	= t{3,3,3}×{ } Truncated 5-cell prism	12	50	100	100	40
62	= rr{3,3,3}×{ } Cantellated 5-cell prism	22	120	250	210	60
63	= t_0,3{3,3,3}×{ } Runcinated 5-cell prism	32	130	200	140	40
64	= 2t{3,3,3}×{ } Bitruncated 5-cell prism	12	60	140	150	60
65	= tr{3,3,3}×{ } Cantitruncated 5-cell prism	22	120	280	300	120
66	= t_0,1,3{3,3,3}×{ } Runcitruncated 5-cell prism	32	180	390	360	120
67	= t_0,1,2,3{3,3,3}×{ } Omnitruncated 5-cell prism	32	210	540	600	240

B₄ × A₁

This prismatic family has 16 forms. (Three are shared with [3,4,3]×[ ] family)

The A₁ x B₄ family has symmetry of order 768 (2*2^4*4!).

#	Coxeter diagram and Schläfli symbols Name	Element counts
#	Coxeter diagram and Schläfli symbols Name	Facets	Cells	Faces	Edges	Vertices
[16]	= {4,3,3}×{ } Tesseractic prism (Same as 5-cube)	10	40	80	80	32
68	= r{4,3,3}×{ } Rectified tesseractic prism	26	136	272	224	64
69	= t{4,3,3}×{ } Truncated tesseractic prism	26	136	304	320	128
70	= rr{4,3,3}×{ } Cantellated tesseractic prism	58	360	784	672	192
71	= t_0,3{4,3,3}×{ } Runcinated tesseractic prism	82	368	608	448	128
72	= 2t{4,3,3}×{ } Bitruncated tesseractic prism	26	168	432	480	192
73	= tr{4,3,3}×{ } Cantitruncated tesseractic prism	58	360	880	960	384
74	= t_0,1,3{4,3,3}×{ } Runcitruncated tesseractic prism	82	528	1216	1152	384
75	= t_0,1,2,3{4,3,3}×{ } Omnitruncated tesseractic prism	82	624	1696	1920	768
76	= {3,3,4}×{ } 16-cell prism	18	64	88	56	16
77	= r{3,3,4}×{ } Rectified 16-cell prism (Same as 24-cell prism)	26	144	288	216	48
78	= t{3,3,4}×{ } Truncated 16-cell prism	26	144	312	288	96
79	= rr{3,3,4}×{ } Cantellated 16-cell prism (Same as rectified 24-cell prism)	50	336	768	672	192
80	= tr{3,3,4}×{ } Cantitruncated 16-cell prism (Same as truncated 24-cell prism)	50	336	864	960	384
81	= t_0,1,3{3,3,4}×{ } Runcitruncated 16-cell prism	82	528	1216	1152	384
82	= sr{3,3,4}×{ } snub 24-cell prism	146	768	1392	960	192

F₄ × A₁

This prismatic family has 10 forms.

The A₁ x F₄ family has symmetry of order 2304 (2*1152). Three polytopes 85,86 and 89 (green background) have double symmetry [[3,4,3],2], order 4608. The last one, snub 24-cell prism, (blue background) has [3⁺,4,3,2] symmetry, order 1152.

#	Coxeter diagram and Schläfli symbols Name	Element counts
#	Coxeter diagram and Schläfli symbols Name	Facets	Cells	Faces	Edges	Vertices
[77]	= {3,4,3}×{ } 24-cell prism	26	144	288	216	48
[79]	= r{3,4,3}×{ } rectified 24-cell prism	50	336	768	672	192
[80]	= t{3,4,3}×{ } truncated 24-cell prism	50	336	864	960	384
83	= rr{3,4,3}×{ } cantellated 24-cell prism	146	1008	2304	2016	576
84	= t_0,3{3,4,3}×{ } runcinated 24-cell prism	242	1152	1920	1296	288
85	= 2t{3,4,3}×{ } bitruncated 24-cell prism	50	432	1248	1440	576
86	= tr{3,4,3}×{ } cantitruncated 24-cell prism	146	1008	2592	2880	1152
87	= t_0,1,3{3,4,3}×{ } runcitruncated 24-cell prism	242	1584	3648	3456	1152
88	= t_0,1,2,3{3,4,3}×{ } omnitruncated 24-cell prism	242	1872	5088	5760	2304
[82]	= s{3,4,3}×{ } snub 24-cell prism	146	768	1392	960	192

H₄ × A₁

This prismatic family has 15 forms:

The A₁ x H₄ family has symmetry of order 28800 (2*14400).

#	Coxeter- diagram and Schläfli symbols Name	Element counts
#	Coxeter- diagram and Schläfli symbols Name	Facets	Cells	Faces	Edges	Vertices
89	= {5,3,3}×{ } 120-cell prism	122	960	2640	3000	1200
90	= r{5,3,3}×{ } Rectified 120-cell prism	722	4560	9840	8400	2400
91	= t{5,3,3}×{ } Truncated 120-cell prism	722	4560	11040	12000	4800
92	= rr{5,3,3}×{ } Cantellated 120-cell prism	1922	12960	29040	25200	7200
93	= t_0,3{5,3,3}×{ } Runcinated 120-cell prism	2642	12720	22080	16800	4800
94	= 2t{5,3,3}×{ } Bitruncated 120-cell prism	722	5760	15840	18000	7200
95	= tr{5,3,3}×{ } Cantitruncated 120-cell prism	1922	12960	32640	36000	14400
96	= t_0,1,3{5,3,3}×{ } Runcitruncated 120-cell prism	2642	18720	44880	43200	14400
97	= t_0,1,2,3{5,3,3}×{ } Omnitruncated 120-cell prism	2642	22320	62880	72000	28800
98	= {3,3,5}×{ } 600-cell prism	602	2400	3120	1560	240
99	= r{3,3,5}×{ } Rectified 600-cell prism	722	5040	10800	7920	1440
100	= t{3,3,5}×{ } Truncated 600-cell prism	722	5040	11520	10080	2880
101	= rr{3,3,5}×{ } Cantellated 600-cell prism	1442	11520	28080	25200	7200
102	= tr{3,3,5}×{ } Cantitruncated 600-cell prism	1442	11520	31680	36000	14400
103	= t_0,1,3{3,3,5}×{ } Runcitruncated 600-cell prism	2642	18720	44880	43200	14400

Grand antiprism prism

The grand antiprism prism is the only known convex non-Wythoffian uniform 5-polytope. It has 200 vertices, 1100 edges, 1940 faces (40 pentagons, 500 squares, 1400 triangles), 1360 cells (600 tetrahedra, 40 pentagonal antiprisms, 700 triangular prisms, 20 pentagonal prisms), and 322 hypercells (2 grand antiprisms , 20 pentagonal antiprism prisms , and 300 tetrahedral prisms ).

#	Name	Element counts
#	Name	Facets	Cells	Faces	Edges	Vertices
104	grand antiprism prism Gappip	322	1360	1940	1100	200

Notes on the Wythoff construction for the uniform 5-polytopes

Construction of the reflective 5-dimensional uniform polytopes are done through a Wythoff construction process, and represented through a Coxeter diagram, where each node represents a mirror. Nodes are ringed to imply which mirrors are active. The full set of uniform polytopes generated are based on the unique permutations of ringed nodes. Uniform 5-polytopes are named in relation to the regular polytopes in each family. Some families have two regular constructors and thus may have two ways of naming them.

Here are the primary operators available for constructing and naming the uniform 5-polytopes.

The last operation, the snub, and more generally the alternation, are the operation that can create nonreflective forms. These are drawn with "hollow rings" at the nodes.

The prismatic forms and bifurcating graphs can use the same truncation indexing notation, but require an explicit numbering system on the nodes for clarity.

Operation	Extended Schläfli symbol		Description
Parent	t₀{p,q,r,s}	{p,q,r,s}	Any regular 5-polytope
Rectified	t₁{p,q,r,s}	r{p,q,r,s}	The edges are fully truncated into single points. The 5-polytope now has the combined faces of the parent and dual.
Birectified	t₂{p,q,r,s}	2r{p,q,r,s}	Birectification reduces faces to points, cells to their duals.
Trirectified	t₃{p,q,r,s}	3r{p,q,r,s}	Trirectification reduces cells to points. (Dual rectification)
Quadrirectified	t₄{p,q,r,s}	4r{p,q,r,s}	Quadrirectification reduces 4-faces to points. (Dual)
Truncated	t_0,1{p,q,r,s}	t{p,q,r,s}	Each original vertex is cut off, with a new face filling the gap. Truncation has a degree of freedom, which has one solution that creates a uniform truncated 5-polytope. The 5-polytope has its original faces doubled in sides, and contains the faces of the dual.
Cantellated	t_0,2{p,q,r,s}	rr{p,q,r,s}	In addition to vertex truncation, each original edge is beveled with new rectangular faces appearing in their place. A uniform cantellation is half way between both the parent and dual forms.
Runcinated	t_0,3{p,q,r,s}		Runcination reduces cells and creates new cells at the vertices and edges.
Stericated	t_0,4{p,q,r,s}	2r2r{p,q,r,s}	Sterication reduces facets and creates new facets (hypercells) at the vertices and edges in the gaps. (Same as expansion operation for 5-polytopes.)
Omnitruncated	t_0,1,2,3,4{p,q,r,s}		All four operators, truncation, cantellation, runcination, and sterication are applied.
Half	h{2p,3,q,r}		Alternation, same as
Cantic	h₂{2p,3,q,r}		Same as
Runcic	h₃{2p,3,q,r}		Same as
Runcicantic	h_2,3{2p,3,q,r}		Same as
Steric	h₄{2p,3,q,r}		Same as
Runcisteric	h_3,4{2p,3,q,r}		Same as
Stericantic	h_2,4{2p,3,q,r}		Same as
Steriruncicantic	h_2,3,4{2p,3,q,r}		Same as
Snub	s{p,2q,r,s}		Alternated truncation
Snub rectified	sr{p,q,2r,s}		Alternated truncated rectification
	ht_0,1,2,3{p,q,r,s}		Alternated runcicantitruncation
Full snub	ht_0,1,2,3,4{p,q,r,s}		Alternated omnitruncation

Regular and uniform honeycombs

There are five fundamental affine Coxeter groups, and 13 prismatic groups that generate regular and uniform tessellations in Euclidean 4-space.^[2]

Fundamental groups
#	Coxeter group			Coxeter diagram	Forms
1	${\tilde {A}}_{4}$	[3^[5]]	[(3,3,3,3,3)]		7
2	${\tilde {C}}_{4}$	[4,3,3,4]			19
3	${\tilde {B}}_{4}$	[4,3,3^1,1]	[4,3,3,4,1⁺]	=	23 (8 new)
4	${\tilde {D}}_{4}$	[3^1,1,1,1]	[1⁺,4,3,3,4,1⁺]	=	9 (0 new)
5	${\tilde {F}}_{4}$	[3,4,3,3]			31 (21 new)

There are three regular honeycombs of Euclidean 4-space:

tesseractic honeycomb, with symbols {4,3,3,4}, = . There are 19 uniform honeycombs in this family.
24-cell honeycomb, with symbols {3,4,3,3}, . There are 31 reflective uniform honeycombs in this family, and one alternated form.
- Truncated 24-cell honeycomb with symbols t{3,4,3,3},
- Snub 24-cell honeycomb, with symbols s{3,4,3,3}, constructed by four snub 24-cell, one 16-cell, and five 5-cells at each vertex.
16-cell honeycomb, with symbols {3,3,4,3},

Other families that generate uniform honeycombs:

There are 23 uniquely ringed forms, 8 new ones in the 16-cell honeycomb family. With symbols h{4,3²,4} it is geometrically identical to the 16-cell honeycomb, =
There are 7 uniquely ringed forms from the ${\tilde {A}}_{4}$ ${\tilde {A}}_{4}$ , family, all new, including:
There are 9 uniquely ringed forms in the ${\tilde {D}}_{4}$ : [3^1,1,1,1] family, two new ones, including the quarter tesseractic honeycomb, = , and the bitruncated tesseractic honeycomb, = .

Non-Wythoffian uniform tessellations in 4-space also exist by elongation (inserting layers), and gyration (rotating layers) from these reflective forms.

Prismatic groups
#	Coxeter group
1	${\tilde {C}}_{3}$ × ${\tilde {I}}_{1}$	[4,3,4,2,∞]
2	${\tilde {B}}_{3}$ × ${\tilde {I}}_{1}$	[4,3^1,1,2,∞]
3	${\tilde {A}}_{3}$ × ${\tilde {I}}_{1}$	[3^[4],2,∞]
4	${\tilde {C}}_{2}$ × ${\tilde {I}}_{1}$ x ${\tilde {I}}_{1}$	[4,4,2,∞,2,∞]
5	${\tilde {H}}_{2}$ × ${\tilde {I}}_{1}$ x ${\tilde {I}}_{1}$	[6,3,2,∞,2,∞]
6	${\tilde {A}}_{2}$ × ${\tilde {I}}_{1}$ x ${\tilde {I}}_{1}$	[3^[3],2,∞,2,∞]
7	${\tilde {I}}_{1}$ × ${\tilde {I}}_{1}$ x ${\tilde {I}}_{1}$ x ${\tilde {I}}_{1}$	[∞,2,∞,2,∞,2,∞]
8	${\tilde {A}}_{2}$ x ${\tilde {A}}_{2}$	[3^[3],2,3^[3]]
9	${\tilde {A}}_{2}$ × ${\tilde {B}}_{2}$	[3^[3],2,4,4]
10	${\tilde {A}}_{2}$ × ${\tilde {G}}_{2}$	[3^[3],2,6,3]
11	${\tilde {B}}_{2}$ × ${\tilde {B}}_{2}$	[4,4,2,4,4]
12	${\tilde {B}}_{2}$ × ${\tilde {G}}_{2}$	[4,4,2,6,3]
13	${\tilde {G}}_{2}$ × ${\tilde {G}}_{2}$	[6,3,2,6,3]

Regular tessellations of hyperbolic 4-space

There are five kinds of convex regular honeycombs and four kinds of star-honeycombs in H⁴ space:^[3]

Honeycomb name	Schläfli Symbol {p,q,r,s}	Facet type {p,q,r}	Cell type {p,q}	Face type {p}	Face figure {s}	Edge figure {r,s}	Vertex figure {q,r,s}	Dual
Order-5 5-cell	{3,3,3,5}	{3,3,3}	{3,3}	{3}	{5}	{3,5}	{3,3,5}	{5,3,3,3}
Order-3 120-cell	{5,3,3,3}	{5,3,3}	{5,3}	{5}	{3}	{3,3}	{3,3,3}	{3,3,3,5}
Order-5 tesseractic	{4,3,3,5}	{4,3,3}	{4,3}	{4}	{5}	{3,5}	{3,3,5}	{5,3,3,4}
Order-4 120-cell	{5,3,3,4}	{5,3,3}	{5,3}	{5}	{4}	{3,4}	{3,3,4}	{4,3,3,5}
Order-5 120-cell	{5,3,3,5}	{5,3,3}	{5,3}	{5}	{5}	{3,5}	{3,3,5}	Self-dual

There are four regular star-honeycombs in H⁴ space:

Honeycomb name	Schläfli Symbol {p,q,r,s}	Facet type {p,q,r}	Cell type {p,q}	Face type {p}	Face figure {s}	Edge figure {r,s}	Vertex figure {q,r,s}	Dual
Order-3 small stellated 120-cell	{5/2,5,3,3}	{5/2,5,3}	{5/2,5}	{5}	{5}	{3,3}	{5,3,3}	{3,3,5,5/2}
Order-5/2 600-cell	{3,3,5,5/2}	{3,3,5}	{3,3}	{3}	{5/2}	{5,5/2}	{3,5,5/2}	{5/2,5,3,3}
Order-5 icosahedral 120-cell	{3,5,5/2,5}	{3,5,5/2}	{3,5}	{3}	{5}	{5/2,5}	{5,5/2,5}	{5,5/2,5,3}
Order-3 great 120-cell	{5,5/2,5,3}	{5,5/2,5}	{5,5/2}	{5}	{3}	{5,3}	{5/2,5,3}	{3,5,5/2,5}

Regular and uniform hyperbolic honeycombs

There are 5 compact hyperbolic Coxeter groups of rank 5, each generating uniform honeycombs in hyperbolic 4-space as permutations of rings of the Coxeter diagrams. There are also 9 paracompact hyperbolic Coxeter groups of rank 5, each generating uniform honeycombs in 4-space as permutations of rings of the Coxeter diagrams. Paracompact groups generate honeycombs with infinite facets or vertex figures.

Compact hyperbolic groups
${\widehat {AF}}_{4}$ = [(3,3,3,3,4)]:	${\bar {DH}}_{4}$ = [5,3,3^1,1]:	${\bar {H}}_{4}$ = [3,3,3,5]: ${\bar {BH}}_{4}$ = [4,3,3,5]: ${\bar {K}}_{4}$ = [5,3,3,5]:

Paracompact hyperbolic groups
${\bar {P}}_{4}$ = [3,3^[4]]: ${\bar {BP}}_{4}$ = [4,3^[4]]: ${\bar {FR}}_{4}$ = [(3,3,4,3,4)]: ${\bar {DP}}_{4}$ = [3^[3]×[]]:	${\bar {N}}_{4}$ = [4,/3\,3,4]: ${\bar {O}}_{4}$ = [3,4,3^1,1]: ${\bar {S}}_{4}$ = [4,3^2,1]: ${\bar {M}}_{4}$ = [4,3^1,1,1]:	${\bar {R}}_{4}$ = [3,4,3,4]:

Notes

References

T. Gosset: On the Regular and Semi-Regular Figures in Space of n Dimensions, Messenger of Mathematics, Macmillan, 1900 (3 regular and one semiregular 4-polytope)
A. Boole Stott: Geometrical deduction of semiregular from regular polytopes and space fillings, Verhandelingen of the Koninklijke academy van Wetenschappen width unit Amsterdam, Eerste Sectie 11,1, Amsterdam, 1910
H.S.M. Coxeter:
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973 (p. 297 Fundamental regions for irreducible groups generated by reflections, Spherical and Euclidean)
- H.S.M. Coxeter, The Beauty of Geometry: Twelve Essays (Chapter 10: Regular honeycombs in hyperbolic space, Summary tables IV p213)
Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [1]
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
- (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591] (p. 287 5D Euclidean groups)
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. Dissertation, University of Toronto, 1966
Klitzing, Richard. "5D uniform polytopes (polytera)".
James E. Humphreys, Reflection Groups and Coxeter Groups, Cambridge studies in advanced mathematics, 29 (1990) (Page 141, 6.9 List of hyperbolic Coxeter groups, figure 2) [2]

External links

Polytope names, Guy Inchbald
Polytopes of Various Dimensions, Jonathan Bowers
Glossary for hyperspace: Polytope, George Olshevsky
Multi-dimensional Glossary, Garrett Jones
polytope names, Wendy Krieger

v t e Fundamental convex regular and uniform polytopes in dimensions 2–10
Family	A_n	B_n	I₂(p) / D_n	E₆ / E₇ / E₈ / F₄ / G₂	H_n
Regular polygon	Triangle	Square	p-gon	Hexagon	Pentagon
Uniform polyhedron	Tetrahedron	Octahedron • Cube	Demicube		Dodecahedron • Icosahedron
Uniform polychoron	Pentachoron	16-cell • Tesseract	Demitesseract	24-cell	120-cell • 600-cell
Uniform 5-polytope	5-simplex	5-orthoplex • 5-cube	5-demicube
Uniform 6-polytope	6-simplex	6-orthoplex • 6-cube	6-demicube	1₂₂ • 2₂₁
Uniform 7-polytope	7-simplex	7-orthoplex • 7-cube	7-demicube	1₃₂ • 2₃₁ • 3₂₁
Uniform 8-polytope	8-simplex	8-orthoplex • 8-cube	8-demicube	1₄₂ • 2₄₁ • 4₂₁
Uniform 9-polytope	9-simplex	9-orthoplex • 9-cube	9-demicube
Uniform 10-polytope	10-simplex	10-orthoplex • 10-cube	10-demicube
Uniform n-polytope	n-simplex	n-orthoplex • n-cube	n-demicube	1_k2 • 2_k1 • k₂₁	n-pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes and compounds

v t e Fundamental convex regular and uniform honeycombs in dimensions 2–9
Space	Family	${\tilde {A}}_{n-1}$	${\tilde {C}}_{n-1}$	${\tilde {B}}_{n-1}$	${\tilde {D}}_{n-1}$	${\tilde {G}}_{2}$ / ${\tilde {F}}_{4}$ / ${\tilde {E}}_{n-1}$
E²	Uniform tiling	{3^[3]}	δ₃	hδ₃	qδ₃	Hexagonal
E³	Uniform convex honeycomb	{3^[4]}	δ₄	hδ₄	qδ₄
E⁴	Uniform 4-honeycomb	{3^[5]}	δ₅	hδ₅	qδ₅	24-cell honeycomb
E⁵	Uniform 5-honeycomb	{3^[6]}	δ₆	hδ₆	qδ₆
E⁶	Uniform 6-honeycomb	{3^[7]}	δ₇	hδ₇	qδ₇	2₂₂
E⁷	Uniform 7-honeycomb	{3^[8]}	δ₈	hδ₈	qδ₈	1₃₃ • 3₃₁
E⁸	Uniform 8-honeycomb	{3^[9]}	δ₉	hδ₉	qδ₉	1₅₂ • 2₅₁ • 5₂₁
E⁹	Uniform 9-honeycomb	{3^[10]}	δ₁₀	hδ₁₀	qδ₁₀
E¹⁰	Uniform 10-honeycomb	{3^[11]}	δ₁₁	hδ₁₁	qδ₁₁
E^n-1	Uniform (n-1)-honeycomb	{3^[n]}	δ_n	hδ_n	qδ_n	1_k2 • 2_k1 • k₂₁

Category:5-polytopes

[1]

[2]

[3]

Search

User:Tomruen/Uniform 5-polytope

Contents

History of discovery

Regular 5-polytopes

Convex uniform 5-polytopes

Reflection families

Enumerating the convex uniform 5-polytopes

The A₅ family

The B₅ family

The D₅ family

Uniform prismatic forms

A₄ × A₁

B₄ × A₁

F₄ × A₁

H₄ × A₁

Grand antiprism prism

Notes on the Wythoff construction for the uniform 5-polytopes

Regular and uniform honeycombs

Regular tessellations of hyperbolic 4-space

Regular and uniform hyperbolic honeycombs

Notes

References

External links

User:Tomruen/Uniform 5-polytope

History of discovery

Regular 5-polytopes

Convex uniform 5-polytopes

Reflection families

Enumerating the convex uniform 5-polytopes

The A5 family

The B5 family

The D5 family

Uniform prismatic forms

A4 × A1

B4 × A1

F4 × A1

H4 × A1

Grand antiprism prism

Notes on the Wythoff construction for the uniform 5-polytopes

Regular and uniform honeycombs

Regular tessellations of hyperbolic 4-space

Regular and uniform hyperbolic honeycombs

Notes

References

External links

The A₅ family

The B₅ family

The D₅ family

A₄ × A₁

B₄ × A₁

F₄ × A₁

H₄ × A₁